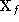 in the space of the structure vectors as the fixation point. Given the
recursively computed scene reconstruction, the transfer of the fixation
point into the new stereopair with computed motion parameters
in the space of the structure vectors as the fixation point. Given the
recursively computed scene reconstruction, the transfer of the fixation
point into the new stereopair with computed motion parameters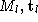 and
and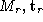 is simply
is simply


The theory of affine transfer presented in [
15
,
5
] is a method of choosing a fixation point on a tracked object, so that
image-plane errors of the fixation point from the centre of the image(s)
may be fed back to the motors controlling the camera(s). In this way a
desired point on the object may be maintained at or near the centre of
the image for as long as the object is tracked. The algorithm involves
constructing a coordinate frame centred on the object, choosing a
fixation point in that coordinate frame, and transferring the point to
new images as they arrive. In the context of the 2D affine stereo
reconstruction algorithm described above, this corresponds to selecting
a point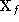 in the space of the structure vectors as the fixation point. Given the
recursively computed scene reconstruction, the transfer of the fixation
point into the new stereopair with computed motion parameters
in the space of the structure vectors as the fixation point. Given the
recursively computed scene reconstruction, the transfer of the fixation
point into the new stereopair with computed motion parameters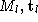 and
and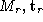 is simply
is simply
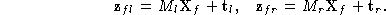
The transferred positions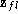 ,
,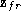 are now converted via triangulation to 3D world coordinates to be
interpreted as a range measurement. It seems sensible to choose the
centroid of the structure vectors computed from the initial batch
computation of the 2D reconstruction for
are now converted via triangulation to 3D world coordinates to be
interpreted as a range measurement. It seems sensible to choose the
centroid of the structure vectors computed from the initial batch
computation of the 2D reconstruction for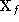 , and this is currently what we do.
, and this is currently what we do.



Adrian F Clark